Addition of Fractions

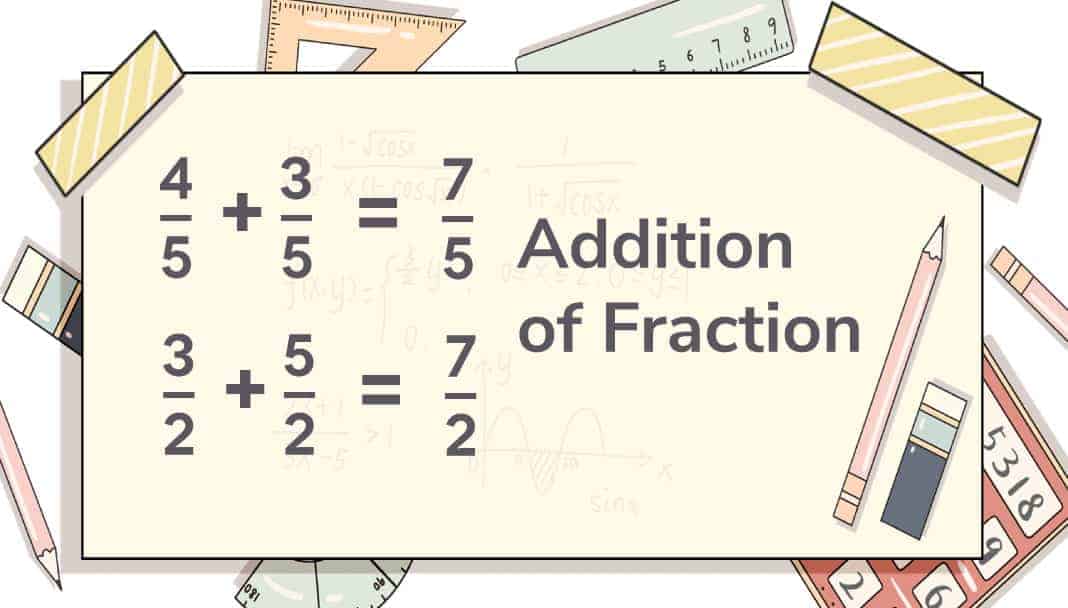
Today we are going to learn about the operations performed on the fraction. Here, we are starting with basic arithmetic operations like addition, and subtraction.

How many methods are used to perform the operations with many examples and practice questions? Hope it will help you to understand the fractions in a better manner.

Let’s start our topic…

Complete Tutorials on Fraction

Part 1: Fractions From Basics
Part 2: Subtraction of Fractions
Part 3: Multiplication of Fractions

Addition & Subtraction of Like and Unlike Fractions

Like  Fraction:

Fractions having the same denominator are referred to as Like Fractions. For eg:- ⅗ and ⅖ or 2/9 and 7/9.

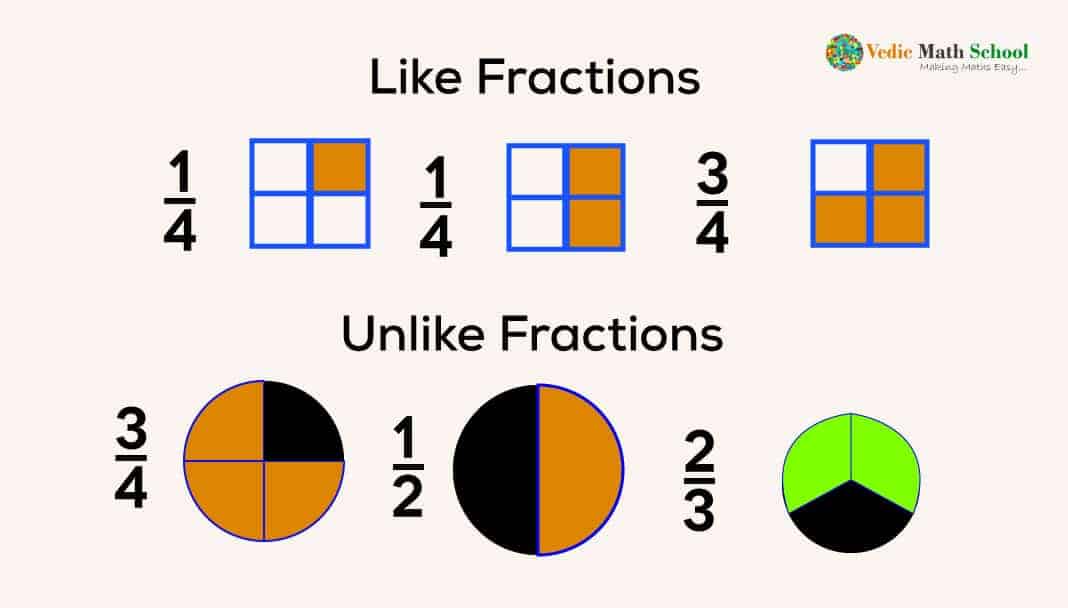
Unlike Fraction

Fractions having different denominators are referred to as, Unlike Fractions. For eg:- ⅗ and ¾  or 2/11 and 7/9.

As discussed earlier, Fraction is a rational number in the form of a/b where b cannot be zero. In a fraction a/b a is known as the numerator and b as the denominator.

For example: –

4/5 is a fraction, where 3 (the upper part) is the numerator and 6 (the lower part) is the denominator.

When we are adding and subtracting fractions, the first thing we have to do is check the denominators, as they will represent the fractions as like and unlike fractions.
We should check if they have the same denominators or different denominators and then the computation starts.

How can we Add and Subtract Fractions?

Addition and subtraction of fractions is not very easy. There are some methods to perform the addition.

The methods are:

1. -Adding fractions having like or the same denominator
2. Adding fractions having unlike or different denominators
3. Adding mixed fractions with the same denominator
4. Adding mixed fractions with different denominators
5. Adding fractions with whole numbers
6. Subtracting fractions having like or the same denominator
7. Subtracting fractions having unlike or different denominator
8. Subtracting mixed fractions with the same denominator
9. Subtracting mixed fractions with different denominators
10. Subtracting fractions from whole numbers

In this module, we will discuss the addition of the fractions with all five methods.

Read More: Remember Multiplication Tables of Any Number Using Mental Mathematics

1. Adding Fractions having like or the same Denominator

We can add the fractions with the same denominator using 3 simple steps. They are: –

Step 1 = Firstly, check if the denominators are the same or not.
Step 2 = If denominators are same, simply add up the numerators.
Step 3 = Now, write the answer with the denominator.

Let us understand these steps more briefly with the help of an example: –

Example 1: 3/5 + 8/5

Step 1 = Check the denominators.
3/5 = denominator is 5.
8/5 = denominator is 5.
So, the denominators are same.

Step 2 = Now, the denominators are the same. Add the numerators.
= 3 + 5 = 8

Step 3 = Write the answer with the denominator.
= 8/5

Example 1: 2/9 + 7/9

Step 1 = Check the denominators.
2/9 = denominator is 9.
7/9 = denominator is 9.
So, the denominators are same.

Step 2 = Now, the denominators are the same. Add the numerators.
= 2 + 7 = 9

Step 3 = Write the answer with the denominator.
= 9/9 = 1

Read More: How to find HCF in 10 seconds

2. Addition of Fractions with Different Denominators

We can easily add fractions with different denominators using a few simple steps. They are: –

Step 1 = Firstly, Find the LCM ( least common multiple) of the denominators.
Step 2 = Now, convert the fractions into equivalent fractions such that their denominators will be the same.
Step 3 = Now, as the denominators are same, simply add on the numerators.
Step 4 = write the answer with the denominator.

Let us understand these steps more briefly with the help of an example: –

Example 1: 3/7 + 1/3

Step 1 = Find the LCM of 7 and 3, which is 21.

Step 2 = Now, convert the fractions into equivalent fractions such that their denominators will be the 21.
= 3/7 * 3/3 = 9/21
= 1/3 * 7/7 = 7/21

Step 3 = The denominators are same now, simply add on the numerators.
= 9 +7 = 16

Step 4 = Write the answer with the denominator.
= 16/21

Example 1: 3/5 + 2/7

Step 1 = Find the LCM of 5 and 7, which is 35.

Step 2 = Now, convert the fractions into equivalent fractions such that their denominators will be 35.
= 3/5 * 7/7 = 21/35
= 2/7 * 5/5 = 10/35

Step 3 = The denominators are the same now, simply add on the numerators.
= 21 + 10 = 31

Step 4 = Write the answer with the denominator.
= 31/35

3. Addition of Mixed Fractions with the same Denominator

We can add the mixed fractions with the same denominator using 3 simple steps. They are: –

Step 1 = Firstly, convert the mixed fractions into improper fractions.
Step 2 = Denominators are the same now, simply add up the numerators.
Step 3 = Now, write the answer with the denominator.

Let us understand these steps more briefly with the help of an example: –

Example 1:

3 ^1/5 +2 ^{1 / 5}

3 ^{1/ 5} + 2 ^{1 / 5}

Step 1 = Convert the mixed fractions into improper fractions

     3 ⅕  = 16/5 when changed to an improper fraction.

Similarly,

     2 ⅕  = 11/5.

Step 2 = Now, the denominators are the same. Add the numerators.
= 16 + 11 = 27

Step 3 = Write the answer with the denominator.
= 27/5

Example 2: 3 ^1/2 + 4 ^{3 / 2}

Step 1 = Convert the mixed fractions into improper fractions.

     3 ½   = 7/2 when changed to improper fraction.

Similarly,

     4 3/2    = 11/2.

Step 2 = Now, the denominators are the same. Add the numerators.
= 7 + 11 = 18

Step 3 = Write the answer with the denominator.
= 18/2 = 9

Read More: Sum of cubes of n natural numbers

4. Addition of Mixed Fractions with Different Denominators 

We can add the mixed fractions with different denominators using a few simple steps. They are: –

Step 1 = Firstly, convert the mixed fractions into improper fractions.
Step 2 = Now, find the LCM ( least common multiple) of the denominators.
Step 3 = Convert the fractions into equivalent fractions such that their denominators will be the same.
Step 4 = Now, as the denominators are the same, simply add on the numerators.
Step 5 = write the answer with the denominator.

Let us understand these steps more briefly with the help of an example: –

Example 1:  2 ^1/3 + 3 ^{3 / 5}

Step 1 = Convert the mixed fractions into improper fractions.
2 ⅓ = 7/3 when changed to an improper fraction.
Similarly,
3 ⅕ = 16/5.

Step 2 = Find the LCM of 3 and 5, which is 15.

Step 3 = Now, convert the fractions into equivalent fractions such that their denominators will be 15.
= 7/3 * 5/5 = 35/15
= 16/5 * 3/3 = 48/15

Step 4 = The denominators are the same now, simply add on the numerators.
= 35 +48 = 83

Example 2: 4 ^1/5 + 3 ^{3 / 7}

Step 1 = Convert the mixed fractions into improper fractions.
4 ⅕ = 21/5 when changed to an improper fraction.
Similarly,
3 1/7 = 22/7.

Step 2 = Find the LCM of 5 and 7, which is 35.

Step 3 = Now, convert the fractions into equivalent fractions such that their denominators will be 15.
= 21/5 * 7/7 = 147/35
= 22/7 * 5/5 = 110/35

Step 4 = The denominators are the same now, simply add on the numerators.
= 147 + 110 = 257

Step 5 = Write the answer with the denominator.
= 257/35

Read More: Basic Concept Of Angles In Geometry

5. Addition of Fractions with Whole Numbers

We can add the fractions with the whole numbers using a few simple steps. They are: –

Step 1 = Firstly, convert the whole number in the form of a/b (fraction) . 3 can be written as 3/1 in a fraction.
Step 2 = Now, find the LCM ( least common multiple) of the denominators.
Step 3 = Convert the fractions into equivalent fractions such that their denominators will be the same.
Step 4 = Now, as the denominators are the same, simply add on the numerators.
Step 5 = write the answer with the denominator.

Let us understand these steps more briefly with the help of an example: –

Example 1: 3 + 2/5

Step 1 = Convert the whole number into fractions.
3 = 3/1 when changed to a fraction..

Step 2 = Now, find the LCM of 1 and 5, which is 5 itself.

Step 3 = Now, convert the fractions into equivalent fractions such that their denominators will be the 5.
= 3/1 * 5/5 = 15/5
= 2/5 * 1/1 = 2/5

Step 4 = The denominators are the same now, simply add on the numerators.
= 15 +02 = 17

Step 5 = Write the answer with the denominator.
= 17/5

Example 2: 7 + 1/4

Step 1 = Convert the whole number into fractions.
7 = 7/1 when changed to a fraction..

Step 2 = Now, find the LCM of 1 and 4, which is 4 itself.

Step 3 = Now, convert the fractions into equivalent fractions such that their denominators will be the 5.
= 7/1 * 4/4 = 28/4
= 1/4 * 1/1 = 1/4

Step 4 = The denominators are the same now, simply add on the numerators.
= 28 +01 = 29

Step 5 = Write the answer with the denominator.
= 29/4

In the next module, we will learn how to subtract the fractions in all five cases.

Read More: Fractions From Basics. Understand What is Fraction, Types with Examples

Practice

1. 2/9 +13/9
2. 35/23 + 12/232/7 + 14/9
3. 7/11 + 3/13
4. 2 ⅕ + 9 ⅕
5. 3 1/7 + 12 2/7
6. 3 ½ + 4 ⅕
7. 2 1/11 + 3 4/13
8. 4 + 2/9
9. 13 + 12/5

Quiz: –

Question 1: What is the sum of ⅖ + ⅗ ?

Options

1. 1 ( correct answer )
2. ⅖
3. ⅗
4. ⅘

Question 2 : what is the sum of ⅗ +4/7 ?

Options

1. 31/35 ( correct answer )
2. 12/7
3. 7/5
4. 1

Question 3 : Which of the following pairs have the same denominator?

Options

1. ⅖ and 3/7
2. 3/7 and 5/7 ( Correct answer )
3. ¼ and 2/8
4. ⅔ and ⅓